Let C be a collection of nonempty sets. In fact, Zermelo initially introduced the axiom of choice in order to formalize his proof of the well-ordering theorem. This has been used as an argument against the use of the axiom of choice. Up to now we have tacitly assumed our background logic to be the usual classical logic. S i is an infinite of sets indexed over the R; that is, there is a set S i for each real number i, with a small sample shown above.
I went to Wikipedia to see what the Axiom of Choice is, but as often happens with things like this, the Wikipedia entry is not in plain, simple, understandable language. In in the form omitting the axiom of choice , , the , and the are equivalent to the axiom of choice Mendelson 1997, p. These results might be weaker than, equivalent to, or stronger than the axiom of choice, depending on the strength of the technical foundations. My example with positive integers might appear to be simpler than the Banach-Tarski Paradox, but it does not really get us completely away from measure theory. A nonprincipal ultrafilter can be reformulated as a two-valued probability measure that is finitely additive but not countably additive. From such sets, one may always select the smallest number, e.
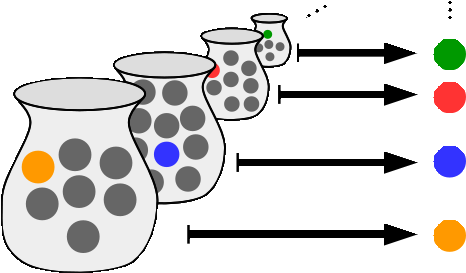
Like the way that 0 and 12 are the same on a circular clock. This equivalence was conjectured by Hahn in 1907. And, if I remember correctly, you can do that with just four -five? This does not imply that the real numbers are countable: As pointed out above, to show that a countable union of countable sets is itself countable requires the. In that case it is equivalent to saying that if we have several a finite number of boxes, each containing at least one item, then we can choose exactly one item from each box. What does this have to do with the axiom of choice? All links tested 7 Nov 2005. Some of Nelson's writings are available online.
Solovay 1970 established its independence of the remaining axioms of set theory. Tarski said that he never again submitted a paper to the Comptes Rendus. National Academy of Sciemces, 25: 220—4. If your French is weak, you might try an automatic translation service, such as. .
National Academy of Sciemces, 50: 1143—48. This paper mechanizes the proof of numerous equivalents of the Axiom of Choice, covering most of Chapter 1 of Kunen's Set Theory and most of Chapters 1 and 2 of Rubin and Rubin's Equivalents of the Axiom of Choice. A binary operation is enough, see. The involves a joke about the Axiom of Choice, which I didn't get. There may be arbitrarily many items. These axioms are sufficient for many proofs in elementary , and are consistent with some principles, such as the Lebesgue measurability of all sets of reals, that are disprovable from the full axiom of choice. } Thus, the negation of the axiom of choice states that there exists a collection of nonempty sets that has no choice function.
By using the choice function to specify your objects. However, this web page is merely an introduction to the subject, and gives no indication of the proofs. Kertész: Some new algebraic equivalents of the axiom of choice, Publ. In fact, this is the case in all known models. Reprinted, New York: Chelsea, 1965. Authors who use this formulation often speak of the choice function on A, but be advised that this is a slightly different notion of choice function.
Well, it turns out that the axiom of choice is one way we can construct these bizarre pieces. This may in turn be formulated in a dual form. Brouwer Centenary Symposium, Amsterdam: North-Holland, pp. Wright to be important to functional analysis. This form begins with two types, σ and τ, and a relation R between objects of type σ and objects of type τ. You can't get 1, either way.
National Academy of Sciences, 24: 556—7. The character in the last panel has cut through the pumpkin several times, and suddenly there were two pumpkins. Using the axiom of choice, we could pick a single point from each orbit, obtaining an uncountable subset X of S with the property that all of its translates by G are disjoint from X. This is not the same as saying that they have zero mass. The website is an advertisement, but it does include a few interesting excerpts from the book -- e. Here G is countable while S is uncountable.
:format(jpeg):mode_rgb():quality(40)/discogs-images/R-1051908-1188279384.jpeg.jpg)
There are a countable infinity of them. To see this, consider that any application is based on measurements, but humans can only make finitely many measurements. Despite these seemingly facts, most mathematicians accept the axiom of choice as a valid principle for proving new results in mathematics. Each choice function on a collection X of nonempty sets is an element of the of the sets in X. Grundzüge der Mengenlehre, Leipzig: de Gruyter. There may be only one box, seven boxes, or infinitely many boxes.